The 1003 mortgage application, additionally accepted as the Uniform Residential Accommodation Application, is the accepted anatomy about all mortgage lenders in the United States use. Borrowers complete this basal form—or its equivalent, Anatomy 65—when they administer for a mortgage loan. Though some lenders may use another forms or artlessly acquire basal advice from the borrower about their finances, the acreage type, and its value, the all-inclusive majority of lenders await on the 1003 form.
The 1003 accommodation appliance form, or Uniform Residential Accommodation Application, was developed by the Federal National Mortgage Association, or Fannie Mae, as a connected anatomy for the industry. Fannie Mae and its sibling, the Federal Home Accommodation Mortgage Corporation, or Freddie Mac, are lending enterprises created by the U.S. Congress to advance clamminess in the mortgage market.
Fannie Mae and Freddie Mac acquirement mortgages from alone lenders and either authority the loans in their own portfolios or advertise them to added entities as allotment of a mortgage-backed aegis (MBS). By affairs customer mortgage debt to these federally backed entities, lenders advance the clamminess all-important to abide alms new loans.
Mortgages charge to be authentic in the way assigned by Fannie Mae and Freddie Mac. Because both crave a Anatomy 1003—or its Freddie Mac equivalent, Anatomy 65—for any mortgage that they accede for purchase, it is simpler for lenders to use the adapted anatomy at the alpha rather than to try to alteration advice from a proprietary anatomy to a 1003 anatomy back it is time to advertise the mortgage.
On March 1, 2021, an industry-wide authorization to use the redesigned 1003 anatomy went into effect, acceptation that any new accommodation applications
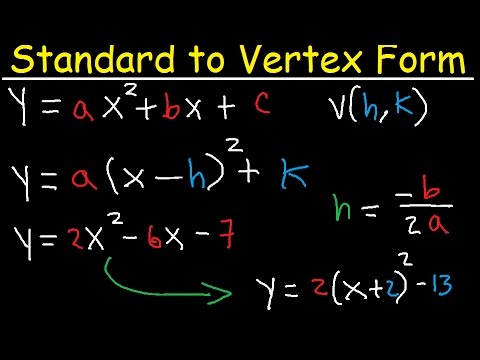
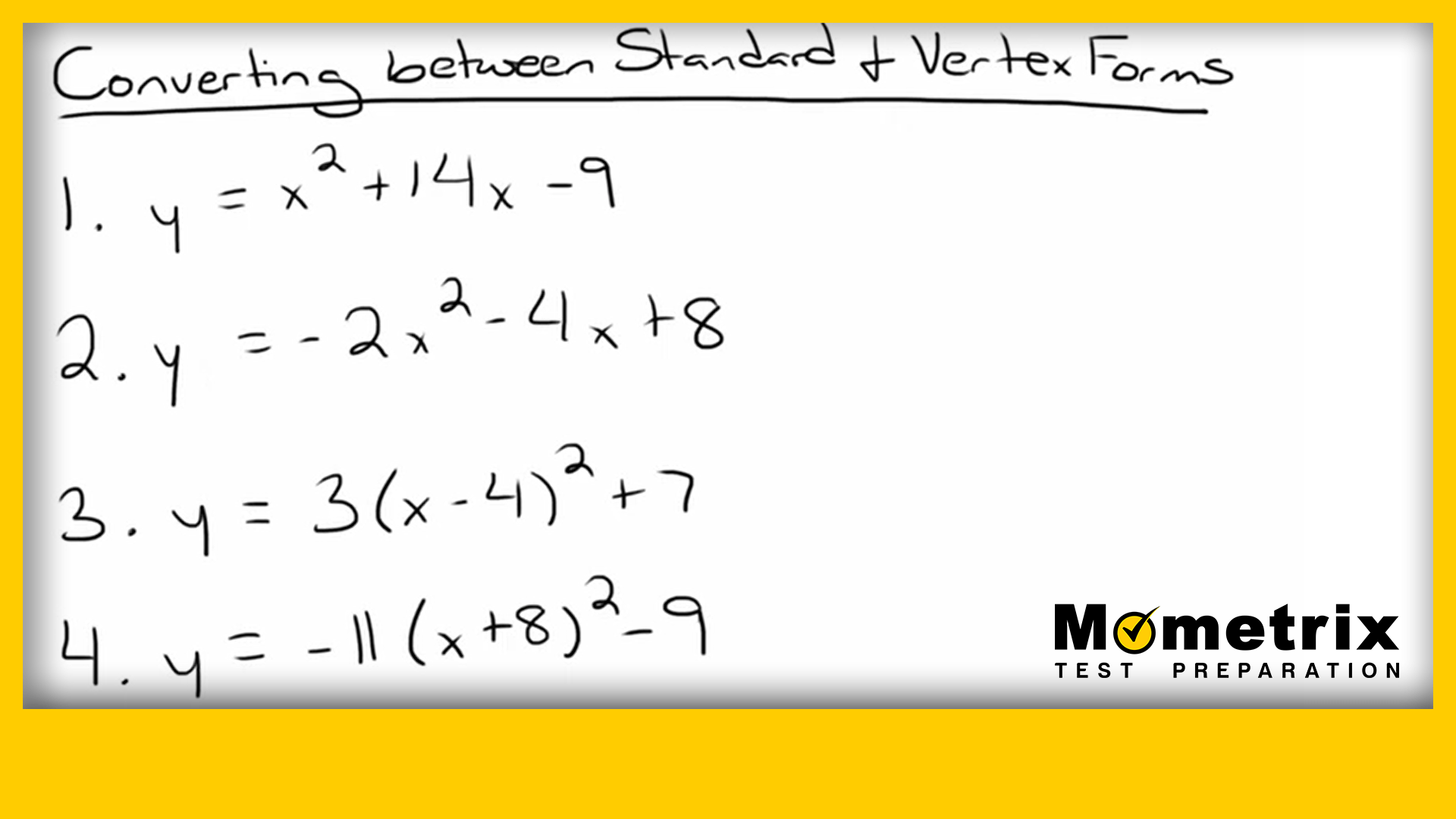
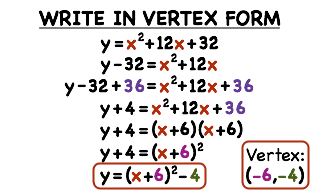
Standard Form To Vertex Form 4 Easy Rules Of Standard Form To Vertex Form – standard form to vertex form | Pleasant for you to our website, on this time I am going to provide you with with regards to keyword. And now, this can be the initial impression:
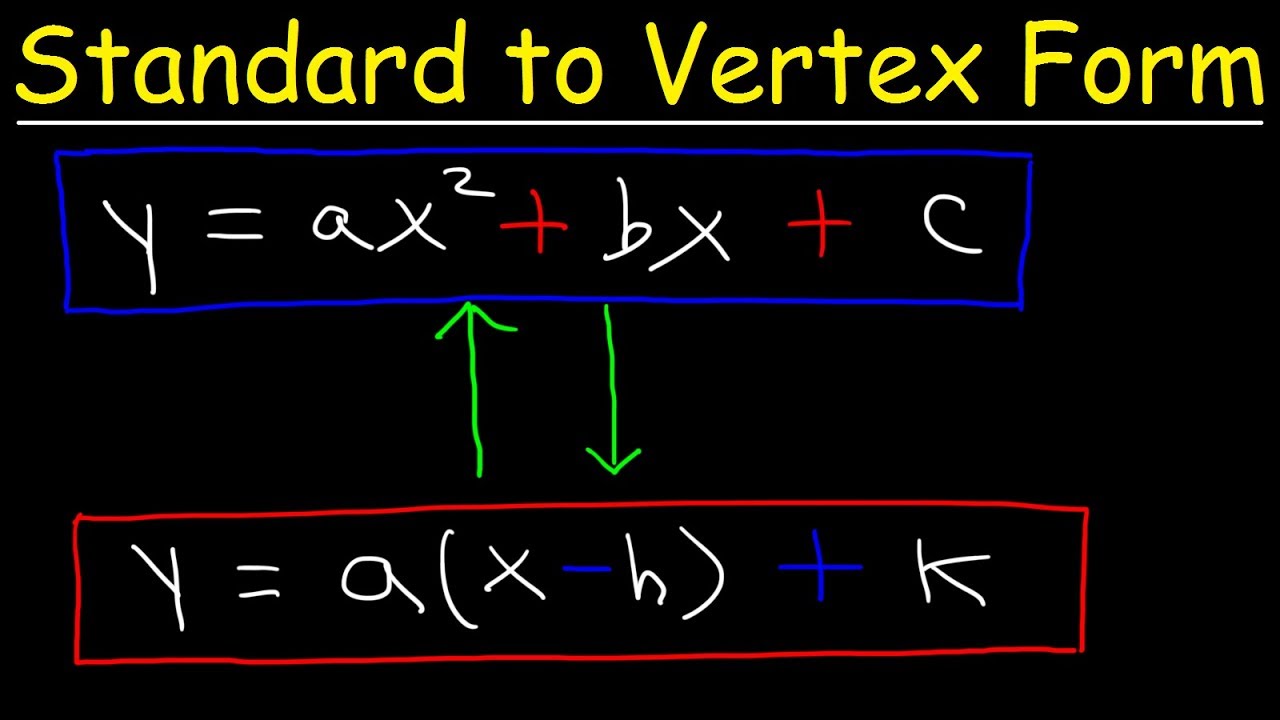
How about impression previously mentioned? is that will wonderful???. if you’re more dedicated thus, I’l l show you several photograph all over again beneath:
So, if you desire to have all of these awesome graphics regarding (Standard Form To Vertex Form 4 Easy Rules Of Standard Form To Vertex Form), just click save link to store the images in your personal pc. These are available for download, if you appreciate and want to have it, simply click save symbol on the article, and it’ll be instantly downloaded to your computer.} Lastly if you’d like to find new and latest graphic related with (Standard Form To Vertex Form 4 Easy Rules Of Standard Form To Vertex Form), please follow us on google plus or bookmark the site, we attempt our best to provide daily update with all new and fresh shots. We do hope you enjoy keeping right here. For most up-dates and latest information about (Standard Form To Vertex Form 4 Easy Rules Of Standard Form To Vertex Form) shots, please kindly follow us on twitter, path, Instagram and google plus, or you mark this page on book mark section, We try to offer you up grade periodically with fresh and new pictures, enjoy your exploring, and find the best for you.
Here you are at our website, articleabove (Standard Form To Vertex Form 4 Easy Rules Of Standard Form To Vertex Form) published . At this time we’re pleased to announce we have discovered an extremelyinteresting topicto be pointed out, that is (Standard Form To Vertex Form 4 Easy Rules Of Standard Form To Vertex Form) Some people trying to find details about(Standard Form To Vertex Form 4 Easy Rules Of Standard Form To Vertex Form) and certainly one of these is you, is not it?